README_EN.md

2033. Minimum Operations to Make a Uni-Value Grid

中文文档

Description

You are given a 2D integer grid of size m x n and an integer x. In one operation, you can add x to or subtract x from any element in the grid.

A uni-value grid is a grid where all the elements of it are equal.

Return the minimum number of operations to make the grid uni-value. If it is not possible, return -1.

 

Example 1:

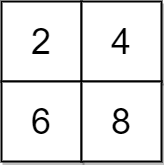
Input: grid = [[2,4],[6,8]], x = 2
Output: 4
Explanation: We can make every element equal to 4 by doing the following: 
- Add x to 2 once.
- Subtract x from 6 once.
- Subtract x from 8 twice.
A total of 4 operations were used.

Example 2:

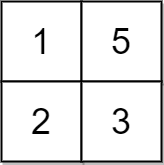
Input: grid = [[1,5],[2,3]], x = 1
Output: 5
Explanation: We can make every element equal to 3.

Example 3:

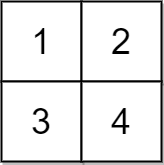
Input: grid = [[1,2],[3,4]], x = 2
Output: -1
Explanation: It is impossible to make every element equal.

 

Constraints:

• m == grid.length
• n == grid[i].length
• 1 <= m, n <= 105
• 1 <= m * n <= 105
• 1 <= x, grid[i][j] <= 104

Solutions

Python3

class Solution:
    def minOperations(self, grid: List[List[int]], x: int) -> int:
        nums = []
        m, n = len(grid), len(grid[0])
        base = grid[0][0]
        for i in range(m):
            for j in range(n):
                if abs(grid[i][j] - base) % x != 0:
                    return -1
                nums.append(grid[i][j])
        nums.sort()
        mid = nums[len(nums) >> 1]
        ans = 0
        for num in nums:
            ans += abs(num - mid) // x
        return ans

Java

class Solution {
    public int minOperations(int[][] grid, int x) {
        int m = grid.length, n = grid[0].length;
        int[] nums = new int[m * n];
        int base = grid[0][0];
        for (int i = 0; i < m; ++i) {
            for (int j = 0; j < n; ++j) {
                if (Math.abs(base - grid[i][j]) % x != 0) {
                    return -1;
                }
                nums[i * n + j] = grid[i][j];
            }
        }
        Arrays.sort(nums);
        int mid = nums[nums.length >> 1];
        int ans = 0;
        for (int num : nums) {
            ans += (Math.abs(num - mid) / x);
        }
        return ans;
    }
}

C++

class Solution {
public:
    int minOperations(vector<vector<int>>& grid, int x) {
        vector<int> nums;
        int m = grid.size(), n = grid[0].size();
        int base = grid[0][0];
        for (int i = 0; i < m; ++i)
        {
            for (int j = 0; j < n; ++j)
            {
                if (abs(grid[i][j] - base) % x != 0) return -1;
                nums.push_back(grid[i][j]);
            }
        }
        sort(nums.begin(), nums.end());
        int mid = nums[nums.size() >> 1];
        int ans = 0;
        for (int num : nums) ans += abs(num - mid) / x;
        return ans;
    }
};

Go

func minOperations(grid [][]int, x int) int {
	var nums []int
	m, n, base := len(grid), len(grid[0]), grid[0][0]
	for i := 0; i < m; i++ {
		for j := 0; j < n; j++ {
			if abs(grid[i][j]-base)%x != 0 {
				return -1
			}
			nums = append(nums, grid[i][j])
		}
	}
	sort.Ints(nums)
	mid := nums[len(nums)>>1]
	ans := 0
	for _, num := range nums {
		ans += abs(num-mid) / x
	}
	return ans
}

func abs(x int) int {
	if x > 0 {
		return x
	}
	return -x
}

...